Screw Symmetry II. Two-One Screw Axis

Course Material Index  Section Index  Previous Page  Next Page

Two-One Screw Axis

For a two-one axis, the amount of translation along the axis is exactly one half of the repeat distance in the lattice along the axis direction. It cannot be any other amount as will be explained now. Consider the molecule labelled A and the effect of a two-one screw axis parallel to, say, the a-axis direction of a crystal as shown in the diagram below:

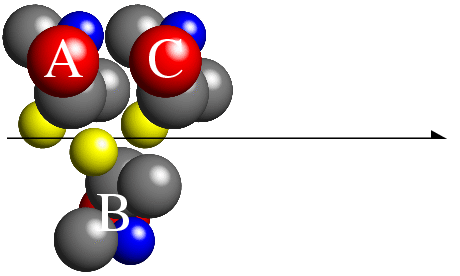
Rotation by 180° about the screw axis followed by a translation of one half of the repeat unit along the a-axis direction moves molecule A to the position of molecule B. This time an atom whose fractional coordinates are x,y,z in molecule A will be related by two-one screw axis symmetry to an atom with fractional coordinates 1/2+x,-y,-z in molecule B.

If this operation is repeated with respect to molecule B, then molecule B moves to the position of molecule C, i.e. the fractional coordinates 1/2+x,-y,-z of the atom in molecule B become 1+x,y,z for the symmetry equivalent atom in molecule C. If the translational component of the screw axis is not exactly 1/2 (of a in this case), the repeated operation of the two-one screw axis will not bring molecule A into exact co-incidence with molecule C.

Hence the operation of a two-one screw axis repeated ad infinitum automatically generates a 1-dimensional lattice along the axis direction as shown in the illustration.

Course Material Index  Section Index  Previous Page  Next Page